SEO Audits & Tools
Get your webpages audited professionally and improve your SEO in minutes.
Paste your URL.
Single page, bulk, sitemap or raw HTML.Run the audit.
Get effective insights in seconds.Fix issues & improve.
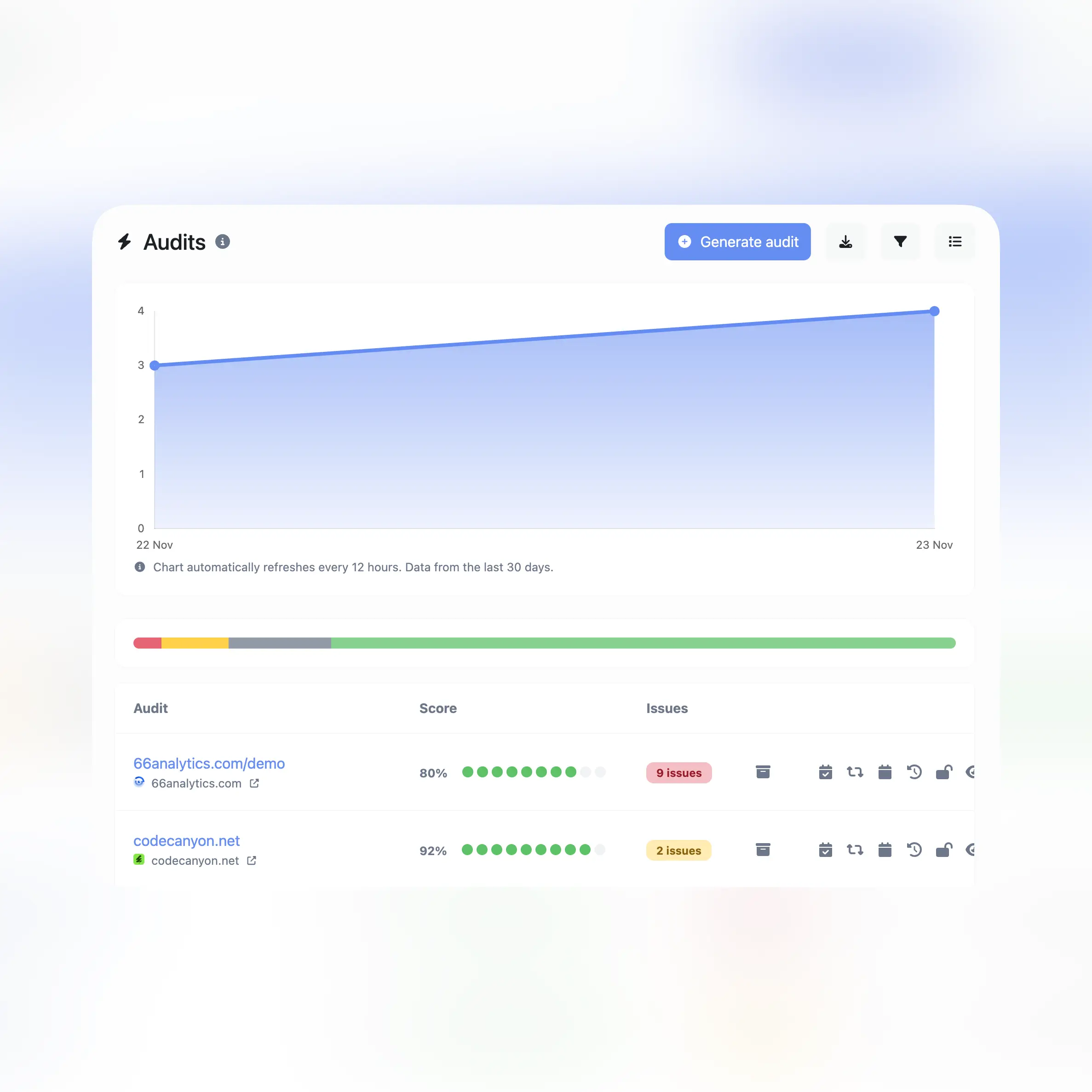
Get actionable tips to improve your SEO
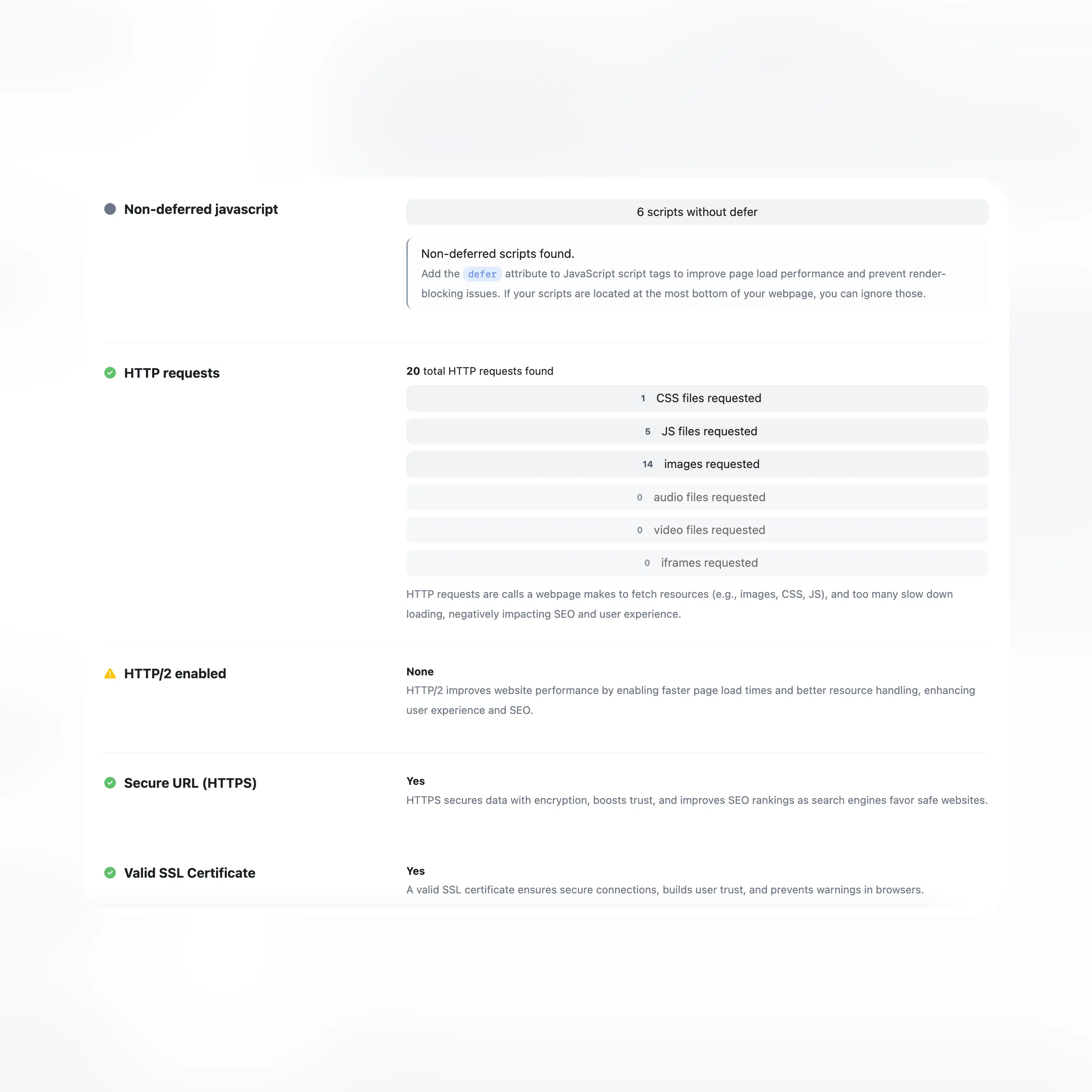
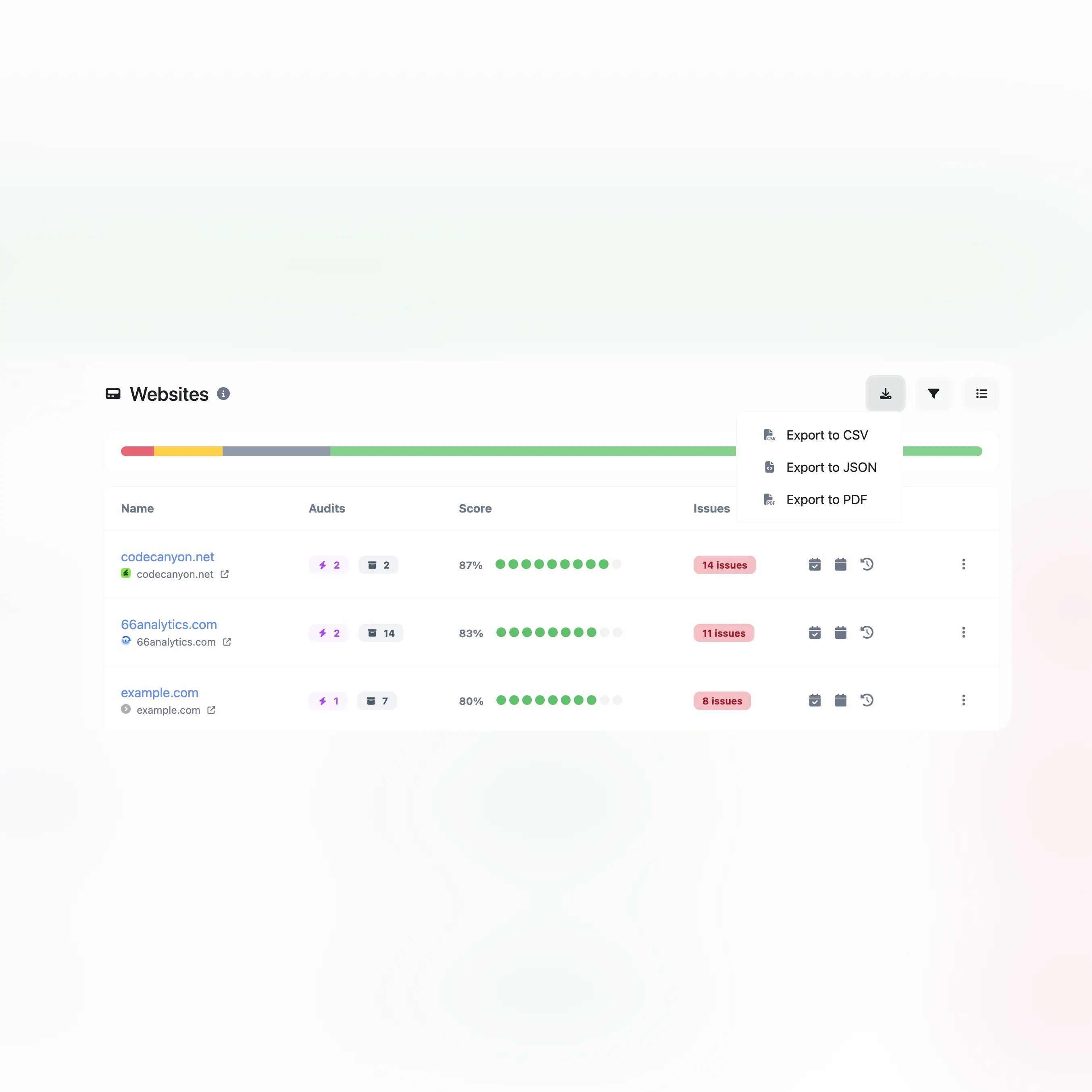
Audit any type of webpage
Versatile, easy to use & modular checks.
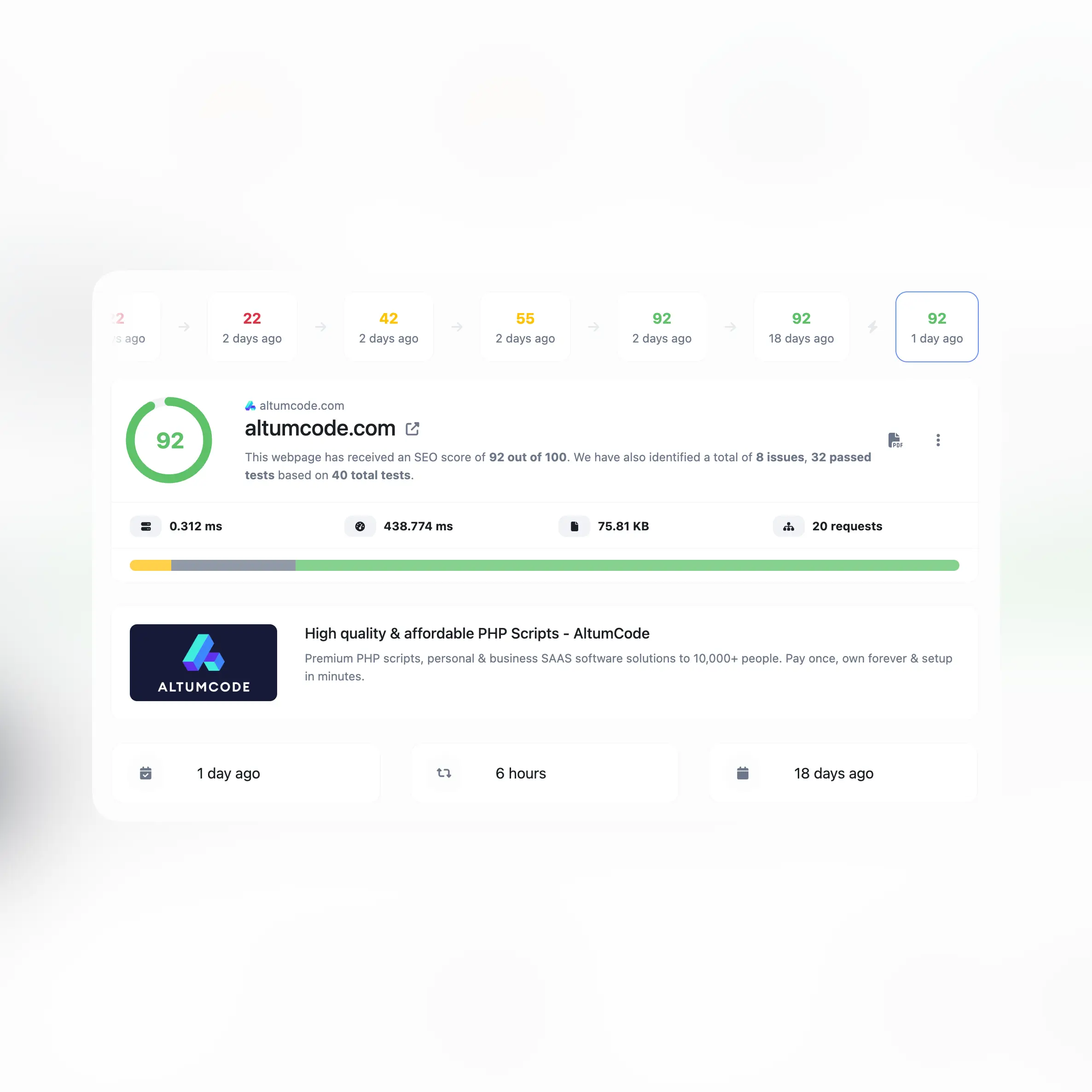
Auditing history
Keep & verify all details of your old audits.
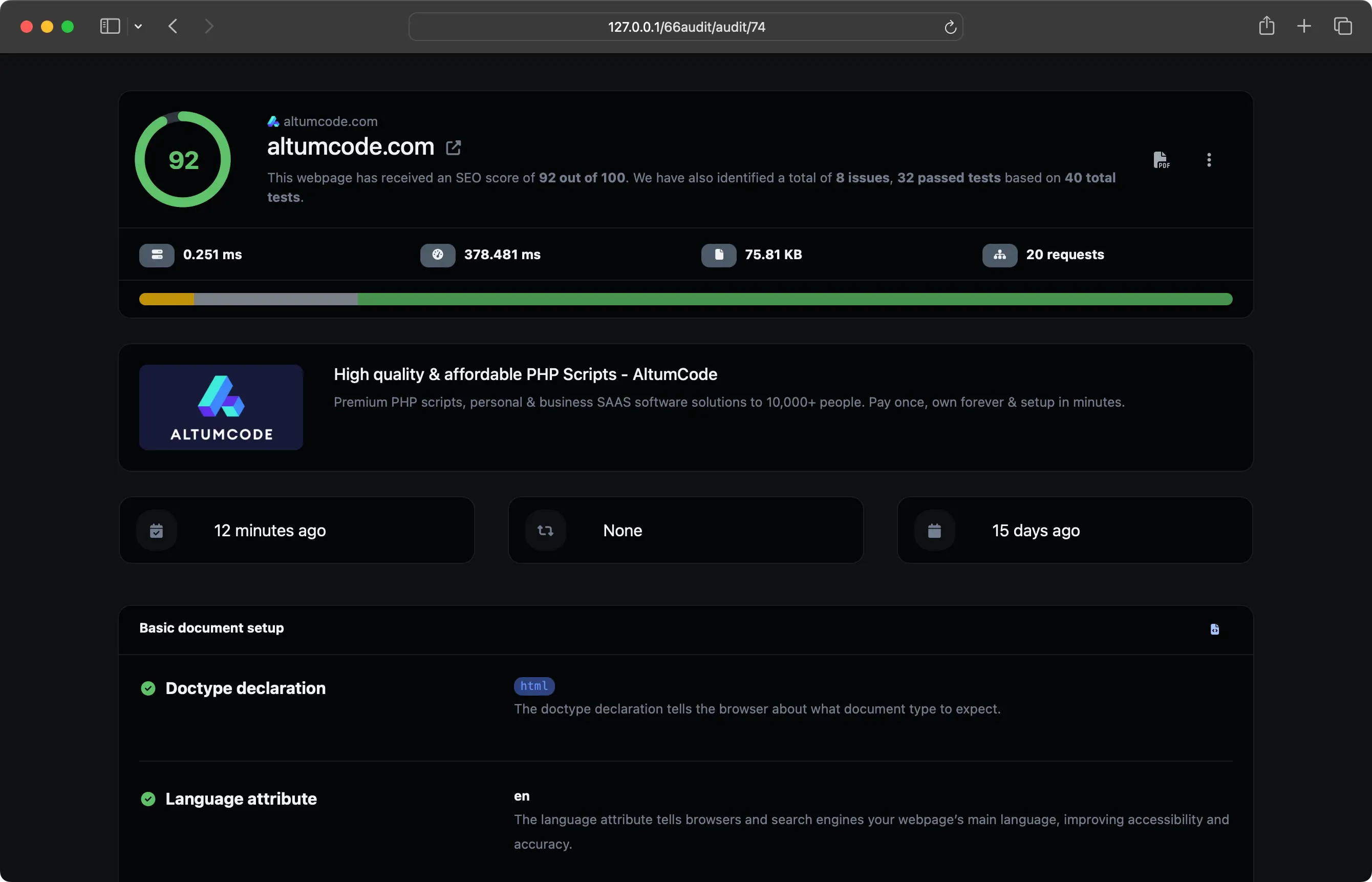
Advanced analysis of SEO
Your complete SEO checklist before going live with a page.
40+ SEO tests
13 additional tools
Web & SEO tools. Fast, reliable and easy to use.
Find A, AAAA, CNAME, MX, NS, TXT, SOA DNS records of a host.
Get approximate IP details.
Get all possible details about an SSL certificate.
Get all possible details about a domain name.
Website status checker.
Get & verify the meta tags of any website.
Get the web-host of a given website.
Get all the HTTP headers that an URL returns for a typical GET request.
Check whether a website is using the new HTTP/2 protocol or not.
Check if the URL is cached or not by Google.
Check for 301 & 302 redirects of a specific URL. It will check for up to 10 redirects.
Take an IP and try to look for the domain/host associated with it.
Check whether a website is using the Brotli Compression algorithm or not.
Get notified on audit refreshes
Countless notification integrations for all your needs.
Here's what people are saying

“ Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ornare ex. Vivamus eu ex non orci condimentum placerat ac ac nisi. Nam velit libero, hendrerit vitae auctor eget, congue ut ante. ”

“ Lorem ipsum dolor sit amet, consectetur adipiscing elit. Praesent finibus vestibulum porta. Sed id eros quam. Nunc at nisi vel arcu placerat gravida. ”

“ Praesent finibus vestibulum porta. Sed id eros quam. Proin lacinia ipsum porttitor, sollicitudin est in, ornare ex. Vivamus eu ex non orci condimentum placerat ac ac nisi. Nam velit libero, hendrerit vitae auctor eget, congue ut ante. ”
Simple, transparent pricing.
Choose the plan that is right for you and your budget.
Answers for your common questions
Fast & accurate.
Get modern & precise audits within seconds.SEO done right.
Powerful technical SEO made easy.We respect your data.
Yours always. Deletable anytime.Get started
Blazing fast SEO audits for webpages.